Domain restriction

Adapted from OpenStax Calculus Volume 1¹

We have seen that $f (x) = x^{2}$ with domain $(- \infty, \infty)$ does not have an inverse function because it is not one-to-one.

graph of y=x^2

However, we can choose a subset of the domain of $f$ such that the function is one-to-one. We call this subset a restricted domain.

For example, we can define new functions $g$ and $h$ such that they both share the same rule as $f$ but have smaller domains. Each of them is one-to-one and has an inverse.

$\begin{aligned} g : x \mapsto x^{2}, & x \in ℝ, x \geq 0, \\ h : x \mapsto x^{2}, & x \in ℝ, x \leq 0 . \end{aligned}$

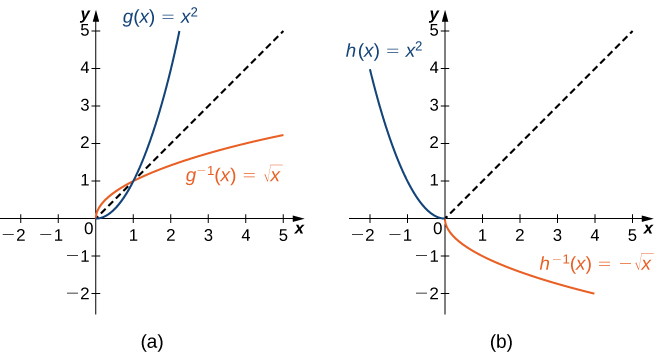
inverses of x^2 under domain restriction

Content in this page is adapted from OpenStax Calculus Volume 1 by Gilbert Strang and Edwin “Jed” Herman under the Creative Commons Attribution Noncommercial Sharealike 4.0 License.
Access for free at https://openstax.org/books/calculus-volume-1/pages/1-4-inverse-functions ↩︎